Page 61 - Contributed Paper Session (CPS) - Volume 8

CPS2184 M Lutfor Rahman
well as cross-sectional version of the dynamic network logistic regression
described in Almquist and Butts (2014).

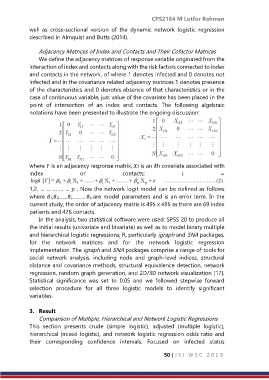
Adjacency Matrices of Index and Contacts and Their Cofactor Matrices
We define the adjacency matrices of response variable originated from the
interaction of index and contacts along with the risk factors connected to index
and contacts in the network, of where 1 denotes infected and 0 denotes not
infected and in the covariance related adjacency matrices 1 denotes presence
of the characteristics and 0 denotes absence of that characteristics or in the
case of continuous variable, just value of the covariate has been placed in the
point of intersection of an index and contacts. The following algebraic
notations have been presented to illustrate the ongoing discussion:

where is an adjacency response matrix, is an ith covariate associated with
index or contacts; =

1,2, … … … … … . Now the network logit model can be defined as follows
where β1,β2,…..,βi,……….βp are model parameters and is an error term. In the
current study, the order of adjacency matrix is 495 x 495 as there are 69 index
patients and 426 contacts.
In the analysis, two statistical software were used: SPSS 20 to produce all
the initial results (univariate and bivariate) as well as to model binary multiple
and hierarchical logistic regressions; R, particularly igraph and SNA packages,
for the network matrices and for the network logistic regression
implementation. The igraph and SNA packages comprise a range of tools for
social network analysis, including node and graph-level indices, structural
distance and covariance methods, structural equivalence detection, network
regression, random graph generation, and 2D/3D network visualization [17].
Statistical significance was set to 0.05 and we followed stepwise forward
selection procedure for all three logistic models to identify significant
variables.

3. Result
Comparison of Multiple, Hierarchical and Network Logistic Regressions
This section presents crude (simple logistic), adjusted (multiple logistic),
hierarchical (mixed logistic), and network logistic regression odds ratio and
their corresponding confidence intervals. Focused on infected status

50 | I S I W S C 2 0 1 9