Page 141 - Contributed Paper Session (CPS) - Volume 3

CPS1972 Livio Corain et al.
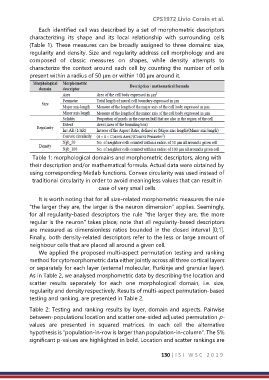
Each identified cell was described by a set of morphometric descriptors
characterizing its shape and its local relationship with surrounding cells
(Table 1). These measures can be broadly assigned to three domains: size,
regularity and density. Size and regularity address cell morphology and are
composed of classic measures on shapes, while density attempts to
characterize the context around each cell by counting the number of cells
present within a radius of 50 μm or within 100 μm around it.

Table 1: morphological domains and morphometric descriptors, along with
their description and/or mathematical formula. Actual data were obtained by
using corresponding Matlab functions. Convex circularity was used instead of
traditional circularity in order to avoid meaningless values that can result in
case of very small cells

It is worth noting that for all size-related morphometric measures the rule
"the larger they are, the larger is the neuron dimension" applies. Seemingly,
for all regularity-based descriptors the rule "the larger they are, the more
regular is the neuron" takes place; note that all regularity-based descriptors
are measured as dimensionless ratios bounded in the closed interval [0;1].
Finally, both density-related descriptors refer to the less or large amount of
neighbour cells that are placed all around a given cell.
We applied the proposed multi-aspect permutation testing and ranking
method for cytomorphometric data either jointly across all three cortical layers
or separately for each layer (external molecular, Purkinje and granular layer).
As in Table 2, we analysed morphometric data by describing the location and
scatter results separately for each one morphological domain, i.e. size,
regularity and density respectively. Results of multi-aspect permutation-based
testing and ranking, are presented in Table 2.

Table 2: Testing and ranking results by layer, domain and aspects. Pairwise
between-populations location and scatter one-sided adjusted permutation p-
values are presented in squared matrices. In each cell the alternative
hypothesis is “population-in-row is larger than population-in-column”. The 5%
significant p-values are highlighted in bold. Location and scatter rankings are

130 | I S I W S C 2 0 1 9