Page 139 - Contributed Paper Session (CPS) - Volume 5

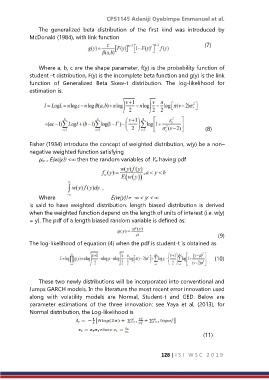
CPS1145 Adeniji Oyebimpe Emmanuel et al.
The generalized beta distribution of the first kind was introduced by
McDonald (1984), with link function
(7)

Where a, b, c are the shape parameter, f(y) is the probability function of
student –t distribution, F(y) is the incomplete beta function and g(y) is the link
function of Generalized Beta Skew-t distribution. The log-likelihood for
estimation is:

(8)

Fisher (1934) introduce the concept of weighted distribution, w(y) be a non–
negative weighted function satisfying
µw = E(w(y)) <∞ then the random variables of Yw having pdf

Where E(w(y))= -∞< y < ∞
is said to have weighted distribution. length biased distribution is derived
when the weighted function depend on the length of units of interest (i.e. w(y)
= y). The pdf of a length biased random variable is defined as:

(9)
The log-likelihood of equation (4) when the pdf is student-t is obtained as

(10)

These two newly distributions will be incorporated into conventional and
Jumps GARCH models. In the literature the most recent error innovation used
along with volatility models are Normal, Student-t and GED. Below are
parameter estimations of the three innovation: see Yaya et al, (2013), for
Normal distribution, the Log-likelihood is

(11)

128 | I S I W S C 2 0 1 9