Page 53 - Contributed Paper Session (CPS) - Volume 3

CPS1943 Nandish C. et al.

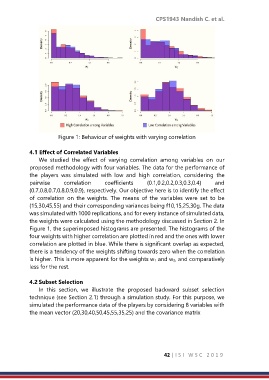
Figure 1: Behaviour of weights with varying correlation

4.1 Effect of Correlated Variables
We studied the effect of varying correlation among variables on our
proposed methodology with four variables. The data for the performance of
the players was simulated with low and high correlation, considering the
pairwise correlation coefficients {0.1,0.2,0.2,0.3,0.3,0.4} and
{0.7,0.8,0.7,0.8,0.9,0.9}, respectively. Our objective here is to identify the effect
of correlation on the weights. The means of the variables were set to be
{15,30,45,55} and their corresponding variances being f10,15,25,30g. The data
was simulated with 1000 replications, and for every instance of simulated data,
the weights were calculated using the methodology discussed in Section 2. In
Figure 1, the superimposed histograms are presented. The histograms of the
four weights with higher correlation are plotted in red and the ones with lower
correlation are plotted in blue. While there is significant overlap as expected,
there is a tendency of the weights shifting towards zero when the correlation
is higher. This is more apparent for the weights w1 and w2, and comparatively
less for the rest.

4.2 Subset Selection
In this section, we illustrate the proposed backward subset selection
technique (see Section 2.1) through a simulation study. For this purpose, we
simulated the performance data of the players by considering 8 variables with
the mean vector (20,30,40,50,45,55,35,25) and the covariance matrix

42 | I S I W S C 2 0 1 9