Page 24 - Contributed Paper Session (CPS) - Volume 8

CPS2151 Sarah B. Balagbis
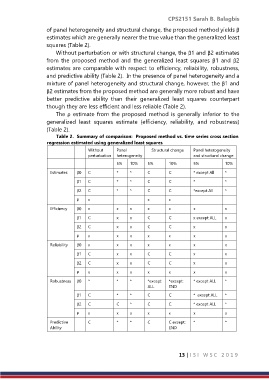
of panel heterogeneity and structural change, the proposed method yields β
estimates which are generally nearer the true value than the generalized least
squares (Table 2).
Without perturbation or with structural change, the β1 and β2 estimates
from the proposed method and the generalized least squares β1 and β2
estimates are comparable with respect to efficiency, reliability, robustness,
and predictive ability (Table 2). In the presence of panel heterogeneity and a
mixture of panel heterogeneity and structural change, however, the β1 and
β2 estimates from the proposed method are generally more robust and have
better predictive ability than their generalized least squares counterpart
though they are less efficient and less reliable (Table 2).
The ρ estimate from the proposed method is generally inferior to the
generalized least squares estimate (efficiency, reliability, and robustness)
(Table 2).
Table 2. Summary of comparison: Proposed method vs. time series cross section
regression estimated using generalized least squares
Without Panel Structural change Panel heterogeneity
perturbation heterogeneity and structural change
5% 10% 5% 10% 5% 10%

Estimates β0 C * * C C * except All *
β1 C * * C C * *
β2 C * * C C *except All *
ρ x x x
Efficiency β0 x x x x x x x

β1 C x x C C x except ALL x
β2 C x x C C x x
ρ x x x x x x x
Reliability β0 x x x x x x x
β1 C x x C C x x
β2 C x x C C x x
ρ x x x x x x x

Robustness β0 * * * *except: *except: * except ALL *
ALL END
β1 C * * C C * except ALL *
β2 C C * C C * except ALL *
ρ x x x x x x x
Predictive C * * C C except: * *
Ability END

13 | I S I W S C 2 0 1 9