Page 22 - Contributed Paper Session (CPS) - Volume 4

CPS2106 Julio M. Singer et al.

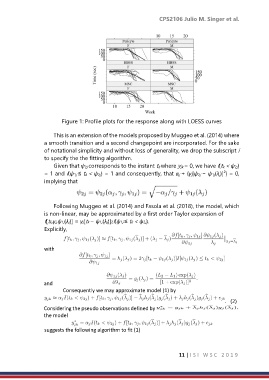
Figure 1: Profile plots for the response along with LOESS curves

This is an extension of the models proposed by Muggeo et al. (2014) where
a smooth transition and a second changepoint are incorporated. For the sake
of notational simplicity and without loss of generality, we drop the subscript i
to specify the the fitting algorithm.
Given that ψ2j corresponds to the instant tk where yjk = 0, we have I(tk < ψ2j)
= 1 and I(ψ1j ≤ tk < ψ2j) = 1 and consequently, that αj + {γj[ψ2j − ψ1j(λj)] } = 0,
2
implying that

Following Muggeo et al. (2014) and Fasola et al. (2018), the model, which
is non-linear, may be approximated by a first order Taylor expansion of
f[tk,γj,ψ1j(λj)] = γj[tk − ψ1j(λj)]2I(ψ1j ≤ tk < ψ2j).
Explicitly,

with

and
Consequently we may approximate model (1) by

. (2)
Considering the pseudo observations defined by
the model

suggests the following algorithm to fit (1)

11 | I S I W S C 2 0 1 9