Page 224 - Contributed Paper Session (CPS) - Volume 4

CPS2201 Mikhail L.
1
Definition 5. Suppose ℱ ′ 4 ⊆ ℱ is a subset of the set of BRDFs. Let Ω , Ω 2
4
sampling strategies be sampling strategies with −uniformly admissible
costs. We say that strategy Ω is asymptotically more efficient for learning
1
2
2
BRDFs of the class ℱ than the strategy Ω , and write Ω < Ω , if
1
′
4

1
′ (( (; Ω ()), ))

(8) lim sup ℱ 4 < 1.
2
→∞ ′ (( (; Ω ()), ))
ℱ 4

Notice that this problem is neither a classification nor a regression task, as
we are picking points to estimate manifolds from noisy data.
A special case of this Definition was used in Langovoy et al. (2016) in order
to propose more efficient BRDF sampling strategies for industrial applications.

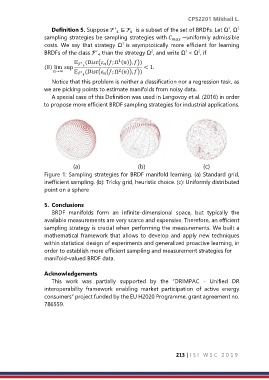
(a) (b) (c)
Figure 1: Sampling strategies for BRDF manifold learning. (a) Standard grid,
inefficient sampling. (b): Tricky grid, heuristic choice. (c): Uniformly distributed
point on a sphere

5. Conclusions
BRDF manifolds form an infinite-dimensional space, but typically the
available measurements are very scarce and expensive. Therefore, an efficient
sampling strategy is crucial when performing the measurements. We built a
mathematical framework that allows to develop and apply new techniques
within statistical design of experiments and generalized proactive learning, in
order to establish more efficient sampling and measurement strategies for
manifold-valued BRDF data.

Acknowledgements
This work was partially supported by the “DRIMPAC - Unified DR
interoperability framework enabling market participation of active energy
consumers” project funded by the EU H2020 Programme, grant agreement no.
786559.

213 | I S I W S C 2 0 1 9