Page 108 - Contributed Paper Session (CPS) - Volume 6

CPS1837 Qiguang Dong et al.
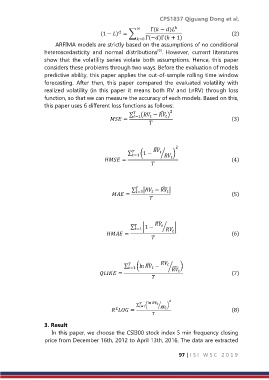
∞ Γ( − )

(1 − ) = ∑ (2)
=0 Γ(−)Γ( + 1)
ARFIMA models are strictly based on the assumptions of no conditional
[9]
heteroscedasticity and normal distributions . However, current literatures
show that the volatility series violate both assumptions. Hence, this paper
considers these problems through two ways. Before the evaluation of models
predictive ability, this paper applies the out-of-sample rolling time window
forecasting. After then, this paper compared the evaluated volatility with
realized volatility (in this paper it means both RV and LnRV) through loss
function, so that we can measure the accuracy of each models. Based on this,
this paper uses 6 different loss functions as follows:
̂
∑ ( − ) 2

=1
= (3)

̂
∑ (1 − ⁄ ) 2
=1
= (4)

̂
∑ | − |
=1

= (5)

̂
∑ |1 − ⁄ |
=1
= (6)

̂
∑ (ln − ⁄ ̂ )
=1

= (7)

2
∑ ( ln ⁄ ̂ )
=1
2
= (8)

3. Result
In this paper, we choose the CSI300 stock index 5 min frequency closing
price from December 16th, 2012 to April 13th, 2016. The data are extracted

97 | I S I W S C 2 0 1 9