Page 188 - Special Topic Session (STS) - Volume 4

STS579 Anastasiia Rytova et al.
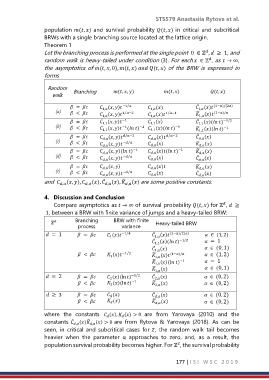
population (, ) and survival probability (, ) in critical and subcritical
BRWs with a single branching source located at the lattice origin.
Theorem 1
Let the branching process is performed at the single point 0 ∈ ℤ , ≥ 1, and

random walk is heavy-tailed under condition (3). For each ∈ ℤ , as → ∞,

the asymptotics of (, , 0), (, ) (, ) of the BRW is expressed in
forms

Random Branching (, , ) (, ) (, )
walk

⁄
= (, ) −1 () ̃ () (1−)/(2)
1,
1,
(a) < (, ) 1 −2 () 1 −1 ̃ () (1−)/
1,
⁄
⁄
1,
1,
1,
= (, ) −1 () ̃ ()( ) −1/2
1,1
1,1
1,1
(b) < (, ) −1 ( ) ()( ) ̃ ()( )
−2
−1
−1
1,1
1,1
1,1
⁄
= (, ) −2 () ⁄ −2 ̃ ()
(c) < , (, ) − , () ̃ , ()
⁄
,
,
,

−1
−1
= , (, )( ) , ()( ) ̃ , ()
(d) < , (, ) − , () ̃ , ()
⁄
= , (, ) , () ̃ , ()
(e) < , (, ) − , () ̃ , ()
⁄

̃
and , (, ), , (), ̃ , (), , () are some positive constants.

4. Discussion and Conclusion

Compare asymptotics as → ∞ of survival probability (, ) for ℤ , ≥
1, between a BRW with finite variance of jumps and a heavy-tailed BRW:
ℤ Branching BRW with finite Heavy-tailed BRW

process variance
= 1 = () −1 4 ̃ () (1−)/(2) ∈ (1, 2)
⁄
1,
1
̃ ()( ) −1/2 = 1
1,1
̃ () ∈ (0, 1)
1,
⁄
< () −1 2 ̃ () (1−)/ ∈ (1, 2)
1
1,
̃ ()( ) = 1
−1
1,1
̃ () ∈ (0, 1)
1,
= 2 = ()(ln ) −1 2 ̃ 2, () ∈ (0, 2)
⁄
2
< ()(ln ) ̃ () ∈ (0, 2)
−1
2
2,

≥ 3 = () ̃ , () ∈ (0, 2)

< () ̃ , () ∈ (0, 2)

where the constants (), () > 0 are from Yarovaya (2010) and the

constants ̃ , () ̃ , () > 0 are from Rytova & Yarovaya (2018). As can be
seen, in critical and subcritical cases for , the random walk tail becomes
heavier when the parameter α approaches to zero, and, as a result, the
population survival probability becomes higher. For ℤ , the survival probability
2
177 | I S I W S C 2 0 1 9