Page 84 - Contributed Paper Session (CPS) - Volume 2

CPS1437 Thanyani M.
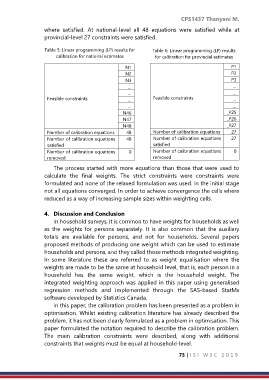
where satisfied. At national-level all 48 equations were satisfied while at
provincial-level 27 constraints were satisfied.

Table 5: Linear programming (LP) results for
calibration for national estimates Table 6: Linear programming (LP) results
for calibration for provincial estimates
N1 P1
N2 P2
N3 P3
… …
… …
Feasible constraints … Feasible constraints …
… …
N46 _P25
N47 _P26
_N48 _P27
Number of calibration equations 48 Number of calibration equations 27
Number of calibration equations 48 Number of calibration equations 27
satisfied satisfied
Number of calibration equations 0 Number of calibration equations 0
removed removed

The process started with more equations than those that were used to
calculate the final weights. The strict constraints were constraints were
formulated and none of the relaxed formulation was used. In the initial stage
not all equations converged. In order to achieve convergence the cells where
reduced as a way of increasing sample sizes within weighting cells.

4. Discussion and Conclusion
In household surveys, it is common to have weights for households as well
as the weights for persons separately. It is also common that the auxiliary
totals are available for persons, and not for households. Several papers
proposed methods of producing one weight which can be used to estimate
households and persons, and they called those methods integrated weighting.
In some literature these are referred to as weight equalisation where the
weights are made to be the same at household level, that is, each person in a
household has the same weight, which is the household weight. The
integrated weighting approach was applied in this paper using generalised
regression methods and implemented through the SAS-based StatMx
software developed by Statistics Canada.
In this paper, the calibration problem has been presented as a problem in
optimisation. Whilst existing calibration literature has already described the
problem, it has not been clearly formulated as a problem in optimisation. This
paper formulated the notation required to describe the calibration problem.
The main calibration constraints were described, along with additional
constraints that weights must be equal at household-level.

73 | I S I W S C 2 0 1 9