Page 131 - Contributed Paper Session (CPS) - Volume 6

CPS1848 J.A. Roldán-Nofuentes et al.
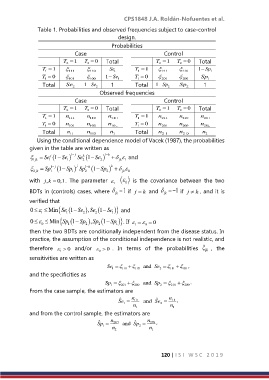
Table 1. Probabilities and observed frequencies subject to case-control
design.
Probabilities
Case Control
T  1 T  0 Total T  1 T  0 Total
2
2
2
2
T  1   Se T  1   1 Sp

1 111 110 1 1 211 210 1
T  0  101  1 Se T  0  201  200 Sp

1
1
1
1
100


Total Se 1 Se 1 Total 1 Sp Sp 1
2
2
2
2
Observed frequencies
Case Control
T  1 T  0 Total T  1 T  0 Total
2
2
2
2
T  1 n 111 n 110 n 11 T  1 n 211 n 210 n 21
1
1
T  0 n n n T  0 n n n
1 101 100 10 1 201 200 20
Total n n 1 0 n Total n 2 1 n 2 0 n
1 1
2
1
Using the conditional dependence model of Vacek (1987), the probabilities
given in the table are written as


 1 jk  Se 1 j 1 Se 1  1 j Se k 2 1 Se 2  1 k    and


1
jk
k
j

 2 jk  Sp 1 j 1 Sp 1  Sp 1 k 1 Sp 2    



1
jk
0
2

with , j k  0,1. The parameter    is the covariance between the two
1 0
BDTs in (controls) cases, where   if j  and    1 if j  , and it is
1
k
k
jk
jk
verified that

0   Min Se 1 1 Se 2 ,Se 2 1 Se 1  and


1


0   Min Sp 1 1 Sp 2 ,Sp 2 1 Sp 1 . If  1   0  0

0
then the two BDTs are conditionally independent from the disease status. In
practice, the assumption of the conditional independence is not realistic, and
therefore   0 and/or   0 . In terms of the probabilities  ijk , the
1 0
sensitivities are written as
Se   111   and Se   111   ,
2
110
101
1
and the specificities as
Sp     and Sp     .
1 201 200 2 210 200
From the case sample, the estimators are
Se  ˆ n 11 and Se  ˆ n 1 1 ,
1 2
n 1 n 1
and from the control sample, the estimators are
Sp  ˆ n 20 and Sp  ˆ n 2 0 ,
1
n 2 n
2 1
120 | I S I W S C 2 0 1 9