Page 124 - Contributed Paper Session (CPS) - Volume 2

CPS1458 KHOO W.C et al.
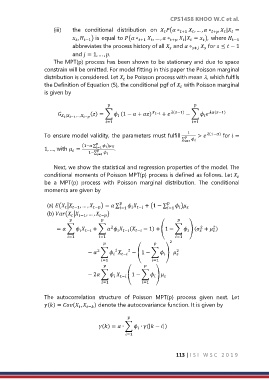
(iii) the conditional distribution on ( ∘ +1 , … , ∘ + | =

, −1 ) is equal to ( ∘ +1 , … , ∘ + | = ), where −1

abbreviates the process history of all and ∘ + for ≤ − 1

and = 1, … , .
The MPT(p) process has been shown to be stationary and due to space
constrain will be omitted. For model fitting in this paper the Poisson marginal
distribution is considered. Let be Poisson process with mean , which fulfils

the Definition of Equation (5), the conditional pgf of with Poisson marginal

is given by

() = ∑ (1 − + ) − + (−1) − ∑ (−1)
| −1 ,…, −
=1 =1

1 (1−)
To ensure model validity, the parameters must fulfill > for =
∑ =1

(1− ∑ )
1, …, with = =1 .

1−∑ =1

Next, we show the statistical and regression properties of the model. The
conditional moments of Poisson MPT(p) process is defined as follows. Let

be a MPT(p) process with Poisson marginal distribution. The conditional
moments are given by

(a) ( | −1 , … , − ) = ∑ + (1 − ∑ )

−
=1
=1
(b) ( | −1 , … , − )

2
2
2
= ∑ + ∑ ( − − 1) + (1 − ∑ ) ( + )

−
−

=1 =1 =1
2
2
2
− ∑ − 2 − (1 − ∑ ) 2

=1 =1

− 2 ∑ − (1 − ∑ )

=1 =1

The autocorrelation structure of Poisson MPT(p) process given next. Let
() = ( , − ) denote the autocovariance function. It is given by

() = ∙ ∑ ∙ (| − |)

=1

113 | I S I W S C 2 0 1 9