Page 78 - Contributed Paper Session (CPS) - Volume 4

CPS2129 Matilde Bini et al.
as latent variables: latent variable means for the intercept and slope factors
describe the averages of initial status and growth rates, respectively; inter-
individual differences in the growth curve parameters are modeled as the
(co)variances of the intercept and slope factors. Given a × 1 vector of
repeated observed measures for individual at time points = 1,2,…,, the
model can be expressed in matrix notation by (Bollen and Curran, 2006): a
trajectory equation, expressed in terms of a confirmatory factor model,
conditional to a vector of time-varying covariates, , in which the latent
factors () represent the growth curve components (intercept and slopes)
= + +

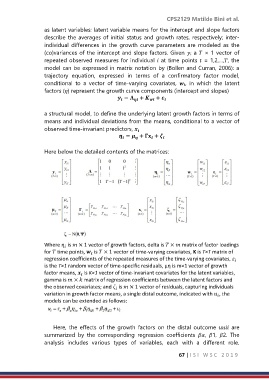
a structural model, to define the underlying latent growth factors in terms of
means and individual deviations from the means, conditional to a vector of
observed time-invariant predictors,

= + +

Here below the detailed contents of the matrices:

Where is × 1 vector of growth factors, delta is × matrix of factor loadings

for time points, is × 1 vector of time-varying covariates, K is T×T matrix of

regression coefficients of the repeated measures of the time-varying covariates,

is the T×1 random vector of time-specific residuals, µη is m×1 vector of growth
factor means, is K×1 vector of time-invariant covariates for the latent variables,

gamma is × matrix of regression coefficients between the latent factors and
the observed covariates; and is × 1 vector of residuals, capturing individuals

variation in growth factor means, a single distal outcome, indicated with , the

models can be extended as follows:

Here, the effects of the growth factors on the distal outcome are
summarized by the corresponding regression coefficients , 1, 2. The
analysis includes various types of variables, each with a different role.

67 | I S I W S C 2 0 1 9