Page 105 - Contributed Paper Session (CPS) - Volume 2

CPS1443 Shogo H.N. et al.
influence of exogenous noise and extract the information of the latent process.
Simple illustration of this idea can be shown as follows: if we denote the
1
̅
̅
̅
sample mean of a sufficiently large number of as , then = + Λ 2 =
ℎ
+ (1) due to [ ] = and Var( ) = . What matters here is the way to
̅

0

0
take the sample mean: when we take the sample mean of the whole
observation, what we have is a -dimensional random variable useless in
estimation. Therefore, it is necessary to separate the observation into a
sufficiently large number of blocks consisting of also a sufficiently large
number of observation. We denote the number of blocks as and that of

samples in each block as such that = , → ∞ and → ∞ as →

∞ . Here the local means , = 0, … , − 1 are defined as the random
̅

variables such that for all = 0, … , − 1,

−1
1
̅
= ∑

Δ +ℎ
=0
where Δ ≔ ℎ indicates the bandwidth of each block with respect to time

such that Δ → 0 as → ∞. Frankly speaking, is the sample mean of
̅

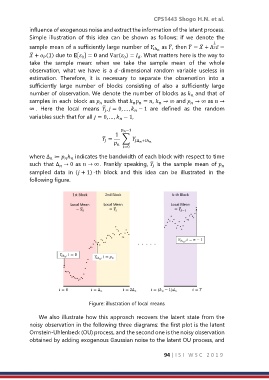
sampled data in ( + 1) -th block and this idea can be illustrated in the
following figure.

Figure: illustration of local means

We also illustrate how this approach recovers the latent state from the
noisy observation in the following three diagrams: the first plot is the latent
Ornstein-Uhlenbeck (OU) process, and the second one is the noisy observation
obtained by adding exogenous Gaussian noise to the latent OU process, and

94 | I S I W S C 2 0 1 9