Page 18 - Contributed Paper Session (CPS) - Volume 3

CPS1923 Deemat C M. et al.
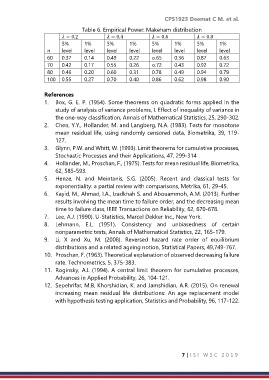
Table 6. Empirical Power: Makeham distribution
= 0.2 = 0.4 = 0.6 = 0.8
5% 1% 5% 1% 5% 1% 5% 1%
level level level level level level level level
60 0.37 0.14 0.49 0.22 o.65 0.36 0.87 0.63
70 0.42 0.17 0.55 0.26 o.72 0.43 0.92 0.72
80 0.46 0.20 0.60 0.31 0.78 0.49 0.94 0.79
100 0.55 0.27 0.70 0.40 0.86 0.62 0.98 0.90

References
1. Box, G. E. P. (1954). Some theorems on quadratic forms applied in the
study of analysis of variance problems, I. Effect of inequality of variance in
the one-way classification, Annals of Mathematical Statistics, 25, 290-302.
2. Chen, Y.Y., Hollander, M. and Langberg, N.A. (1983). Tests for monotone
mean residual life, using randomly censored data, Biometrika, 39, 119-
127.
3. Glynn, P.W. and Whitt, W. (1993). Limit theorems for cumulative processes,
Stochastic Processes and their Applications, 47, 299-314
4. Hollander, M., Proschan, F., (1975). Tests for mean residual life, Biometrika,
62, 585-593.
5. Henze, N. and Meintanis, S.G. (2005). Recent and classical tests for
exponentiality: a partial review with comparisons, Metrika, 61, 29-45.
6. Kayid, M., Ahmad, I.A., Izadkhah S. and Abouammoh, A.M. (2013). Further
results involving the mean time to failure order, and the decreasing mean
time to failure class, IEEE Transactions on Reliability, 62, 670-678.
7. Lee, A.J. (1990). U-Statistics, Marcel Dekker Inc., New York.
8. Lehmann, E.L. (1951). Consistency and unbiasedness of certain
nonparametric tests, Annals of Mathematical Statistics, 22, 165-179.
9. Li, X and Xu, M. (2008). Reversed hazard rate order of equilibrium
distributions and a related ageing notion, Statistical Papers, 49,749-767.
10. Proschan, F. (1963). Theoretical explanation of observed decreasing failure
rate. Technometrics, 5, 375-383.
11. Roginsky, A.L (1994). A central limit theorem for cumulative processes,
Advances in Applied Probability, 26, 104-121.
12. Sepehrifar, M.B, Khorshidian, K. and Jamshidian, A.R. (2015). On renewal
increasing mean residual life distributions: An age replacement model
with hypothesis testing application, Statistics and Probability, 96, 117-122.

7 | I S I W S C 2 0 1 9