Page 67 - Contributed Paper Session (CPS) - Volume 2

CPS1428 Enobong F. U.
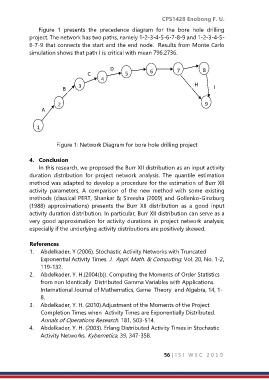
Figure 1 presents the precedence diagram for the bore hole drilling
project. The network has two paths, namely 1-2-3-4-5-6-7-8-9 and 1-2-3-4-5-
6-7-9 that connects the start and the end node. Results from Monte Carlo
simulation shows that path I is critical with mean 796.2736.

D 7 8
C 5 6
4
3 H
B I

2 9
A

1

Figure 1: Network Diagram for bore hole drilling project

4. Conclusion
In this research, we proposed the Burr XII distribution as an input activity
duration distribution for project network analysis. The quantile estimation
method was adapted to develop a procedure for the estimation of Burr XII
activity parameters. A comparison of the new method with some existing
methods (classical PERT, Shankar & Sireesha (2009) and Gollenko-Ginzburg
(1988) approximations) presents the Burr XII distribution as a good input
activity duration distribution. In particular, Burr XII distribution can serve as a
very good approximation for activity durations in project network analysis;
especially if the underlying activity distributions are positively skewed.

References
1. Abdelkader, Y (2006). Stochastic Activity Networks with Truncated
Exponential Activity Times. J. Appl. Math. & Computing. Vol. 20, No. 1-2,
119-132.
2. Abdelkader, Y. H.(2004(b)). Computing the Moments of Order Statistics
from non Identically Distributed Gamma Variables with Applications.
International Journal of Mathematics, Game Theory and Algebra, 14, 1-
8.
3. Abdelkader, Y. H. (2010).Adjustment of the Moments of the Project
Completion Times when Activity Times are Exponentially Distributed.
Annals of Operations Research. 181, 503-514.
4. Abdelkader, Y. H. (2003). Erlang Distributed Activity Times in Stochastic
Activity Networks. Kybernetica, 39, 347-358.

56 | I S I W S C 2 0 1 9